Particle-wave duality: Difference between revisions
No edit summary |
No edit summary |
||
| Line 3: | Line 3: | ||
To be more specific on the wave nature of matter, a particle moving with constant velocity, \(v\), can be ascribed a corresponding (de-Broglie) wavelength, given by | To be more specific on the wave nature of matter, a particle moving with constant velocity, \(v\), can be ascribed a corresponding (de-Broglie) wavelength, given by | ||
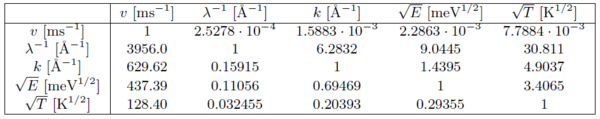
<figtable id="tab:conversion">[[File: | <figtable id="tab:conversion">[[File:Tabel1.1.PNG| thumb | 600px |<caption>Conversion table between different neutron parameters in the most commonly used units. Examples of use: \( v \, [{\rm ms}^{-1}] = 629.6 \, k \, [\)Å\(^{-1}]\) and \( (\lambda[\)Å\(])^{-1} = 0.1106 \sqrt{E {\rm [meV]}} \).</caption>]]</figtable> | ||
\begin{equation}\label{dummy1979310179} | \begin{equation}\label{dummy1979310179} | ||
Latest revision as of 11:28, 24 March 2020
One of the remarkable consequences of quantum mechanics is that matter has both particle- and wave-like nature[1]. The neutron is no exception from this. In neutron scattering experiments, neutrons behave predominantly as particles when they are created in a nuclear process, as interfering waves when they are scattered, and again as particles when they are detected by another nuclear process.
To be more specific on the wave nature of matter, a particle moving with constant velocity, \(v\), can be ascribed a corresponding (de-Broglie) wavelength, given by
\begin{equation}\label{dummy1979310179} \lambda = \dfrac{2 \pi \hbar}{m v} . \end{equation}
In neutron scattering, the wave nature is often referred to in terms of the neutron wave number,
\begin{equation}\label{dummy1979310179321321} k = \frac{2 \pi}{\lambda} , \end{equation}
or the wave vector of length \(k\) and with same direction as the velocity:
\begin{equation}\label{eq:wavenumber} \mathbf{k} = \dfrac{m_{\rm n} \mathbf{v}}{\hbar} . \end{equation}
By tradition, wavelengths are measured in Å (\(10^{-10} {\rm \,m}\)), and wave numbers in Å\({}^{-1}\), although some groups rather tend to use nm and nm\({}^{-1}\). The neutron velocity is always measured in SI units: m/s. For our purpose we consider the neutrons as non-relativistic, and the neutron kinetic energy is given by
\begin{equation}\label{dummy1797195180} E = \dfrac{\hbar^2 k^2}{2 m_{\rm n}} , \end{equation}
which is measured in eV or meV, where \(1 {\rm eV} = 1.60218 \cdot 10^{-19} {\rm \,J}\). A useful conversion table between velocity, wave number, wavelength, energy, and temperature, is shown in Table xx--CrossReference--tab:conversion--xx[2].