Neutron guide systems

The earliest neutron scattering instruments used a beam of neutrons, extracted from the moderator through holes (or tunnels) in the shielding; also called a beam port. The neutron intensity from this type of beam port falls off in general as \(1/r^2\); for details: see the problem The beam port. This square law dependence dictates that neutron instruments of this type are placed close to the neutron source. Therefore they will suffer from a relatively high background from the source, e.g. from gamma-radiation, and from epithermal and fast neutrons.

In the early 1960's, a new concept was invented: The neutron guide. This is a neutron conducting channel, in principle equivalent to an optical fiber. The guide can extract a beam of neutrons from the moderator and deliver it at another point, further away from the neutron source. Typical guide lengths are 10-100 m.

We will here take a closer look into neutron guide systems.

Guide reflectivity

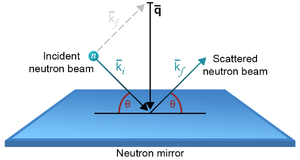
The neutron guide builds on the principle that surfaces of materials with positive values of the scattering length \(b\) show total reflection of thermal and cold neutrons under sufficiently small angles. At larger angles, the reflectivity falls off to zero very fast.

Figure 1: Illustration of the geometry of a neutron reflecting off the surface of a guide, also known as a neutron mirror.

We define the critical angle, \(\theta_{\rm c}(\lambda)\), as the largest angle between the neutron path and the surface that still gives rise to total reflection. For a given material, the critical angle is proportional to the neutron wavelength. Following the equation for \(q\) from \(k\) and \(\theta\) and the corresponding figure from the Basics of neutron scattering page, the critical scattering vector is now given by

\begin{equation}\label{dummy613884281} q_{\rm c} = 2 k \sin(\theta_{\rm c} \,(\lambda)) \approx 4 \pi \dfrac{\theta_{\rm c}(\lambda)}{\lambda} , \end{equation}

when \(\theta_{\rm c}\) is given in radians. In general, \(q_{\rm c}\) is independent of \(\lambda\). For the standard guide material, Ni, the critical scattering vector is

\begin{equation}\label{dummy339440531} q_{\rm c, Ni} = 0.0217 {\rm Å}^{-1} . \end{equation}

Modern guides are made from multilayer material, usually with Ni as the outmost layer. This ensures total reflectivity up to \({q}_{\rm c, Ni}\). In addition, the reflectivity is non-zero up to a much higher scattering vector

\begin{equation}\label{dummy799289935} {q}_{\rm c} = {m q}_{\rm c, Ni} .\, \end{equation}

One therefore often speaks about the \(m\)-value of a multilayer, e.g. an \(m=3\) guide. An example of a reflectivity profile for an $m=3.6$ guide is shown in Figure xx--CrossReference--fig:guide_R--xx (right). Typical values of \(m\) are 2-4, although mirrors with up to \(m=7\) can now be obtained. Multilayer guides can be purchased commercially, typically in pieces of 0.5 m length and cross sections of up to \(300 \times 300\) mm\(^2\).

Experimental considerations

For neutrons of 1~Å and 10~Å wavelengths, the critical angles from Ni become \(\theta_{\rm c} = \eta_{\rm x} = 0.100^{\circ}\) and \(1.00^{\circ}\), respectively. This should be compared with the divergence requirements from present instruments, which is often in the range \(0.2^{\circ}\) to \(1^{\circ}\) Hence, neutron optics for 10~Å neutrons is a fairly easy task that can be performed with Ni mirrors, while shorter wavelengths present increasingly larger challenges, where higher \(m\)-value supermirrors are needed.

Straight guides

The "classical" and most often used guide system is the straight guide, where the guide cross section is constant along the full length of the guide. Typical sizes of cross sections from \(20 \times 20\) mm\(^2\) to \(120 \times 30\) mm\(^2\). Assuming the guide to be sufficiently long, the maximum divergence being transported through the guide is

\begin{equation}\label{dummy421761879} \theta_c = \eta_x = 0.100^\circ m \frac{\lambda}{[\mbox{Å}]}, \end{equation}

and the same for the \(y\)-direction. However, the effect of the supermirror decreases for large divergences (larger than \(\theta_{\rm c}\)), since neutrons will typically experience many reflections, and thus be attenuated by the reflectivity value to a high power.

In addition, we notice that the maximal volume of the available phase space in the guide is proportional to \(m^2 \lambda^2\). This explains why, until recently, guides were primarily used for cold neutrons and thermal instruments were most often placed at a beam port close to the moderator.

Curved guides

In practice, many constant-cross-section guides have sections which are slightly curved horizontally, with radii of the order \(R \approx 0.5-3\) km, while keeping the constant guide cross section. This is done in order to avoid direct line-of-sight from the moderator to the experiment, strongly reducing the number of hot and epithermal neutrons passing down the guide, which in turn minimizes the experimental background.

For an example of this guide cut-off, imagine a neutron which bounces alternatingly off the left and right walls of a guide with width \(w\). The guide curves to the left with the radius of curvature \(R_{\rm c}\). We consider the limiting case, where the neutron just glances off the left wall, \(2\theta = 0\), which gives the smallest possible scattering angle on the following reflection on the wall. Due to the curvature, the neutron will hit the right wall at an angle \(\theta = \sqrt{2w/R}\), corresponding to a scattering vector of \(q=(4 \pi/\lambda) \sqrt{2w/R}\). To scatter the neutron, we need \(q \leq m q_{\rm c, Ni}\). This leads to a condition for the zig-zag scattered neutrons

\begin{equation}\label{dummy1778420237} \lambda \geq \dfrac{4\pi}{mq_{\rm c,Ni}} \sqrt{\dfrac{2w}{R}} . \end{equation}

Using the typical values for a cold-neutron guide: \(m=2\), \(w=30\) mm, and \(R=2400\) m, we reach a lower cut-off for the transmitted neutrons: \(\lambda \geq 1.435\) Å. However, this is not the whole truth. Neutron paths exist, where the neutrons repeatedly scatter off the outer (in this case the left) wall only. The phase space of these so-called garland reflections trajectories is, however, much smaller than the regular left-right trajectories.

One way to overcome the garland reflections is to bend the guide first in one direction, then in the opposite. In this so-called S-shaped guide, the shortest wavelengths are completely eliminated.

Tapering guides

To increase the intensity of neutrons onto small samples, some existing guide systems have recently been equipped with focusing noses, which are often a linear (or curving) tapering piece of high-\(m\) supermirror guide that narrows the beam just before the sample. A well-designed nose will increase the number of neutrons hitting the sample, but according to the Liouville theorem, this comes at the expense of an increased beam divergence. In addition, the divergence profile from a tapering nose is often non-uniform, due to the difference in divergence between neutrons hitting the nose piece zero, one, or more times, respectively.

A full guide set-up using tapering guides is often known as a ballistic guide system. The guide starts with a linearly expanding section, followed by a straight (possibly curved) section, to end with a tapering converging nose. This guide system has the advantage that the expanding section decreases the divergence of the transmitted beam (at the cost of a decreased spatial density, according to Liouville). The lower divergence decreases the number of reflections and hence improves the guide transmission. At the nose piece, the neutron density again increases, with a corresponding increase of divergence .

Parabolic and elliptical guides

The parabolic guide system is an improved version of the ballistic guide. It consists of a parabolic expanding start, which ideally (for a point source) would make the beam completely parallel. Then follows a straight (possibly curved) section, and the guide system ends with a parabolic nose, which compresses the beam onto the sample. For practical reasons, the guide is often parabolic in the \(x\) and \(y\) directions separately, so that the cross section of the guide is at any place rectangular.

The elliptical guide system consists of one fully elliptical piece with the moderator at (or close to) one focal point and the sample close to the other focal point. Ideally (for a point source), each neutron would then be reflected only once between moderator and sample, although recent work has shown that a finite size of the source would result in more than one reflection. How simple it may sound, elliptical guides are only just being installed at the first instruments, with very good results, e.g. at the cold-neutron diffractometer WISH at ISIS. In practice, also here, the guide cross section is rectangular, so that a general neutron would need a reflection both in the \(x\) and the \(y\)-direction.

Recent simulation work has shown that parabolic and elliptical guides have almost equal neutron transport properties over large distances (50 m and above), and that they outperform any other guide systems with transmissions of the phase space density close to the Liouville limit. However, there is yet no detailed experimental evidence for this result.

Shielding and shutters

To avoid neutron background outside the guide, a neutron absorbing material is being used to shield its outside. In addition, the gamma radiation produced by the neutron capture is shielded, using (typically) lead, steel, or concrete.

For safety reasons, the neutron beam can be blocked close to the guide entry by a primary beam shutter, which will be closed during long-term maintenance. The secondary beam shutter is placed at the guide end, close before the sample, and will be used for minor experimental interruptions, like change of sample. These shutters are made by a combination of materials that absorb both neutrons and gamma radiation.