Inelastic magnetic scattering
This chapter is devoted to inelastic neutron scattering from magnetic systems, which is one of the hallmarks of neutron scattering. For the investigation of magnetic excitations, neutron scattering is simply the technique of choice.
We begin with a general description of excitations in magnetic materials. Later sections contain a general description of the neutron scattering from magnetic excitations, including cross sections for ferro- and antiferromagnetic spinwaves, magnetic dynamics in nanoparticles, and scattering from quantum spin systems.
*Magnetic excitations
Here we discuss the coherent magnetic excitations known as spin waves. The first section deals with spin waves in a ferromagnet, then we extend the discussion to the much more difficult antiferromagnetic spin waves. We end the chapter by touching the topic of quantum magnets.
*Spin waves in a ferromagnet
Let us assume that the system can be described by a Heisenberg Hamiltonian of the form shown on the Elastic magnetic scattering page.
We assume that the sign of the interaction, \(J_{\delta}\), is dominantly positive; i.e. that the Fourier transform of the spin-spin interaction, \(J({\bf q}')\), is maximal at \(q'=0\). (\(J(\bf q)\) is defined in this equation on the Magnetic neutron scattering page.) In that case, \({\bf Q} = 0\), the ground state of the system is the ferromagnet
\begin{equation} \label{dummy791517836} | GS \rangle = | {\rm FM} \rangle = | \uparrow\uparrow\uparrow\uparrow \rangle , \end{equation}
where all spins point along the positive \(z\)-axis. This quantization axis is in practice defined by the direction of an applied field or some small anisotropy terms, not present in Heisenberg form. We will here neglect anisotropies and just assume that a quantization axis exists. We will describe the elementary excitations in the ferromagnet in two ways: The equation-of-motion method and the stationary operator method, both of which are described below.
Solving the Hamiltonian (\ref{eq:Heisenberg}) quantum mechanically is an easy task for a ferromagnet. Let us first rewrite the spin product term: \begin{equation} {\bf S}_i \cdot {\bf S}_j = s_i^z s_j^ z + \frac{1}{2} (s_i^+ s_j^- + s_i^- s_j^+) , \end{equation} where the spin raising and lowering operators are defined as \begin{align} \label{eq:spinraising} s_{j}^+ &= s_{j}^x + i s_{j}^y , \\ s_{j}^- &= s_{j}^x - i s_{j}^y . \end{align} The raising/lowering operators have the effect of changing \(m\), the eigenvalue of \(s^z\) for one spin, by one unit: \begin{align} s_{j}^+ |S,m\rangle_j &= \sqrt{(S-m)(S+m+1)} |m+1\rangle_j , \\ s_{j}^- |S,m\rangle_j &= \sqrt{(S-m+1)(S+m)} |m-1\rangle_j . \end{align}
We can see that when using the Hamiltonian (\ref{eq:Heisenberg}) on the ferromagnetic state, \(|{\rm FM}\rangle = |\uparrow \uparrow \uparrow \uparrow \rangle\), all spin terms containing \(s^+\) vanish, leaving only the diagonal \(s^z_i s^z_j\) terms. \begin{equation} H | {\rm FM} \rangle = - \sum_{\langle ij \rangle} J_{ij} S^2 | {\rm FM} \rangle . \end{equation} The ferromagnetic state is thus an eigenstate of the Hamiltonian, and is also the ground state. However, for antiferromagnetic couplings, (\ref{eq:Heisenberg}) is a very difficult many-body problem. This can be seen from just considering the two-spin model for spins \(S=1/2\). Here, the classical ground states are \(| {\rm AFM}_1 \rangle = | \uparrow \downarrow \rangle\) and \(| {\rm AFM}_2 \rangle = | \downarrow \uparrow \rangle\). None of these states are eigenstates to (\ref{eq:Heisenberg}), since terms in the Hamiltonian of the type \(s_1^+ s_2^-\) mix the states: \begin{align} H | {\rm AFM}_1 \rangle &= - \frac{J}{4} |{\rm AFM}_1 \rangle + \frac{J}{2} |{\rm AFM}_2 \rangle , \\ H | {\rm AFM}_2 \rangle &= + \frac{J}{2} |{\rm AFM}_1 \rangle - \frac{J}{4} |{\rm AFM}_2 \rangle . \end{align} The resulting ground state is a spin singlet: \begin{equation} |{\rm GS}\rangle = \frac{1}{\sqrt{2}} \left({|\rm AFM}_1 \rangle - |{\rm AFM}_2 \rangle \right) , \end{equation} with a ground state energy of \(-3J/4\), three times lower than (the expectation value of) the energy of the classical solution.
*The equation-of-motion method
Spin excitation frequencies can in general be determined by the ``equation-of-motion approach \cite{marshall,Blundell}. We assume that the spin components perpendicular to the spin ordering \(z\)-direction, \(s_j^x(t)\) and \(s_j^y(t)\), can be non-zero, although their time average is zero. We shall then show that these perpendicular components will perform periodic motion.
To find the time dependence of the perpendicular components, one must take into account that the spins are angular momenta. Hence, a field (or interaction) trying to align the spin along the \(z\)-axis, instead causes the spins to precess around this axis. We could now write down the classical equations of motion for the spin system, but it turns out to be mathematically simpler to perform a quantum mechanical treatment, which we will therefore do.
We start by considering the spin component along the ordering direction, \(s_j^z(t)\). For the equation of motion of this spin operator, we use the Ehrenfest theorem:
\begin{equation} i \hbar \frac{d}{dt} s_j^z = [s_j^z, \hat{H}] \end{equation} to reach \begin{align} \label{eq:fm_motion_z} i \hbar \frac{d}{dt} s_j^z &= \sum_{j'} \left[s_j^z, J({\bf r}_j-{\bf r}_{j'}) (s_j^x s_{j'}^x + s_j^y s_{j'}^y)\right] \nonumber \\ &= \sum_{j'} J({\bf r}_j-{\bf r}_{j'}) \left(s_{j'}^x s_j^y - s_{j'}^y s_j^x \right) \approx 0. \end{align} We have in the derivation neglected commutator terms that immediately vanish. The last approximation to zero is valid since the terms inside the sum are all small to second order - and in fact can be shown to have a time average of exactly zero.
Thus, the spin value along the ordering directions is a constant of motion.
Next, we consider the linear combination \(s_j^+(t) = s_j^x(t) + i s_j^y(t)\), also known as the spin raising operator, defined in (\ref{eq:spinraising}). The Ehrenfest theorem yields:
\begin{align} \label{eq:fm_motion} i \hbar \frac{d}{dt} s_j^+ &= [s_j^+,\hat{H}] \nonumber \\ &= \sum_{j'} \left[(s_j^x + i s_j^y), J({\bf r}_j-{\bf r}_{j'}) (s_j^x s_{j'}^x + s_j^y s_{j'}^y + s_j^z s_{j'}^z - g \mu_{\rm B} B s_j^z )\right] \nonumber \\ &= g \mu_{\rm B} B s_j^+ + \sum_{j'} J({\bf r}_j-{\bf r}_{j'}) \left(s_{j'}^z s_j^+ - s_{j'}^+ s_j^z \right) . \end{align}
As often in solid state physics, we turn to the Fourier transform; this time of the spin operators \begin{equation} S_{\bf q'}^+ = \frac{1}{\sqrt{N}} \sum_j \exp(i {\bf q}'\cdot {\bf r}_j) s_j^+ , \end{equation} where \(N\) is the number of spins. Combined with (\ref{eq:fm_motion}), we arrive at \begin{equation} \label{eq:fm_motion2} \hbar \frac{d}{dt} S_{\bf q'}^+ = g \mu_{\rm B} B S_{\bf q'}^+ + \frac{1}{\sqrt{N}} \sum_{j,j'} \exp(-i {\bf q}'\cdot{\bf r}_j) J({\bf r}_j-{\bf r}_{j'}) \left(s_{j'}^z s_j^+ - s_{j'}^+ s_j^z \right) . \end{equation} Now, in the ground state, the operator \(s_j^z\) has the eigenvalue \(S\). At finite temperatures, we approximate this operator by its thermal mean value, the ordered moment \(\langle S^z \rangle\). Hence, we simplify (\ref{eq:fm_motion2}) \begin{align} \hbar \frac{d}{dt}S_{\bf q'}^+ &= g \mu_{\rm B} B S_{\bf q'}^+ + \frac{\langle S^z \rangle}{\sqrt{N}} \sum_{j,j'} \exp(i {\bf q}'\cdot{\bf r}_j) J({\bf r}_j-{\bf r}_{j'}) \left(s_j^+ - s_{j'}^+ \right) \nonumber \\ &= \big( g \mu_{\rm B} B + \langle S^z \rangle [J({\bf 0})-J({\bf q}')] \big) S_{\bf q'}^+ . \end{align} This differential equation shows that \(S_{\bf q'}^+\) performs a periodic motion with the frequency \begin{equation} \label{eq:fm_omegaq} \hbar\omega_{\bf q'} = g \mu_{\rm B} B + \langle S \rangle [J({\bf 0})-J({\bf q}')] . \end{equation} Now, let us investigate the significance of this result. Assume that \(S_{\bf q'}^+\) is the annihilation operator of one elementary excitation of the system, \begin{equation} S_{\bf q'}^+ |\bf q'\rangle = |{\rm GS}\rangle , \end{equation} where the energy of the groundstate is \(E_0\) and of the excited state \(| {\bf q}'\rangle\) is denoted \(E_0 + \hbar \omega_{\bf q'}\). The time dependent operator, \(S_{\bf q'}^+(t)\), is then found directly by \begin{equation} S_{\bf q'}^+(t) | {\bf q}' \rangle = \exp\left( \frac{i H t}{\hbar} \right) S_{\bf q'}^+ \exp\left( - \frac{i H t}{\hbar} \right) | {\bf q}' \rangle = \exp(-i \omega_{\rm q'} t) S_{\bf q'}^+ | {\bf q'} \rangle . \end{equation} Hence, \(\omega_{\bf q'}\) is the frequency of the spin deviation, and \(\hbar \omega_{\bf q'}\) is the energy of the elementary (spin wave) excitation of the system.
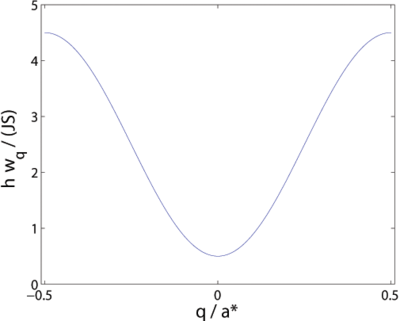
\paragraph{Example.} For nearest neighbour interactions in one dimension, each spin interacts only with its two neighbours. Hence \(J(q') = J(0) \cos(q'd)\), and \(J(0) = 2J\), giving \begin{equation} \label{eq:omegaq_1Dnn} \hbar \omega_{\bf q'} = g \mu_{\rm B} B + 2 J S \big( 1-\cos(q'd) \big) . \end{equation} Hence, for small values of \(|q'|\) (compared to the reciprocal lattice vector, \(2\pi/d\)), the spin wave energy in zero field becomes quadratic in \(q'\). Furthermore, it can be seen that an applied field induces an energy gap of \(\Delta = g \mu_{\rm B} B\). These effects are illustrated in Figure xx--CrossReference--fig:omegaq_FM--xx.
*The stationary operator method
If the ground state can be described (or approximated) in simple terms, it is in general possible to describe the lowest excited states by exact quantum mechanical calculations. For the ferromagnet, this is particularly simple. Let us first define the lowering operator in reciprocal space as
\begin{equation} \label{dummy1791072476} S_{\mathbf q}^- = \dfrac{1}{\sqrt{N}} \displaystyle\sum_j \exp(i {\mathbf q}\cdot {\mathbf r}_j) s_j^- . \end{equation}
It can be shown by direct application of the Hamiltonian form that the state
\begin{equation} \label{dummy1472779595} |{\mathbf q}\rangle = S_{\mathbf q}^- |0\rangle \end{equation}
is an eigenstate of the Hamiltonian,
\begin{equation} \label{dummy1594344458} \hat{H}|{\mathbf q}\rangle = (E_0 + \hbar\omega_{\mathbf q})|{\mathbf q}\rangle. \end{equation}
Reassuringly, for zero temperature \((\langle S^z \rangle = S)\) this gives the same spin wave frequency, \(\omega_{\bf q}\), as the equation-of-motion method in equation \eqref{eq:fm_omegaq}.
*Spin waves in an antiferromagnet
We now proceed to the much more difficult task of calculating the spin waves in a two-sublattice antiferromagnet (AFM), as first described by P. W. Anderson[1]. We will use the equation-of-motion method as described in the section The equation-of-motion method.
The anisotropic Hamiltonian
We assume that the ground state for the AFM is the classical Neél state, where the spins are fully aligned along the positive \(z\)-axis (sublattice m) or along the negative \(z\)-axis (sublattice n), as described in the section Magnetism in materials on the Magnetic neutron scattering page.
We write the magnetic Hamiltonian as an isotropic Heisenberg term, an applied field, \(B\), and an effective anisotropy field, \(B_{\rm A}\), originating from the surrounding crystal. Both fields are parallel to the \(z\)-axis[2].
\begin{align}\label{dummy1663920410} \hat{H} &= \displaystyle\sum_{\mathbf m,r} J({\mathbf r}) {\mathbf s}_{\mathbf m}\cdot{\mathbf s}_{\mathbf m+r} + \displaystyle\sum_{\mathbf n,r} J({\mathbf r}) {\mathbf s}_{\mathbf n}\cdot{\mathbf s}_{\mathbf n+r} \\ &\quad+ \displaystyle\sum_{\mathbf m,R} J_1({\mathbf R}) {\mathbf s}_{\mathbf m}\cdot{\mathbf s}_{\mathbf m+R} + \displaystyle\sum_{\mathbf n,R} J_1({\mathbf R}) {\mathbf s}_{\mathbf n}\cdot{\mathbf s}_{\mathbf n+R} \nonumber\\ &\quad- g \mu_{\rm B} \left(B+B_{\rm A}\right) \displaystyle\sum_{\mathbf m} s_{\mathbf m}^z - g \mu_{\rm B} \left(B-B_{\rm A}\right) \displaystyle\sum_{\mathbf n} s_{\mathbf n}^z ,\nonumber \end{align}
where \({\bf r}\) connects spins on the same sublattice with coupling strength \(J({\bf r})\), and \({\bf R}\) connects spins on opposite sublattices with coupling \(J_1({\bf R})\). Note that \({\bf B}_{\rm A}\) points in opposite directions in the two sublattices, because the anisotropy is uniaxial, i.e. it aims the spin to be parallel to a certain direction, but does not have preference to one of the two directions.
*The equations of motion
We now simplify the description by transforming the spin operators on the "down" sublattice (n) by
\begin{equation}\label{dummy1097282024} s_{\rm n}^x = t_{\rm n}^x , \quad s_{\rm n}^y = - t_{\rm n}^y , \quad s_{\rm n}^z = - t_{\rm n}^z . \end{equation}
As a start, we recall the commutation relations (\ref{eq:commute_pm}): \begin{align} \label{eq:commute_pm} [ s_j^+ , s_{j'}^- ] &= 2 s_j^z \delta_{j,j'} \approx 2 \langle S \rangle \delta_{j,j'} , \\ [ s_j^z , s_{j'}^\pm ] &= \pm s_j^\pm \delta_{j,j'} , \\ [ s_j^x , s_{j'}^y ] &= i s_j^z \delta_{j,j'} \; {\rm and\, cyclic\, permutations.} \end{align}
where \(\langle S \rangle\) is here the ordered moment of the sublattice. This important approximation is of course valid only when the Neél state is a good approximation to the actual state of the system. We now calculate the equations of motion, keeping only terms linear in the operators:
\begin{align} i \hbar \dfrac{d}{dt}s_{\mathbf m}^+ &= \left[ s_{\mathbf m} , \hat{H} \right] \label{dummy390816099}\\ &\stackrel{\rm (1st\, order)}{=} 2 S \displaystyle\sum_{\mathbf r} J({\mathbf r}) (s_{\mathbf m}^+ + t_{\mathbf m+r}^-) \nonumber\\ &\quad+ 2 S \displaystyle\sum_{\mathbf R} J_1({\mathbf R}) (-s_{\mathbf m}^+ + s_{\mathbf m+R}^+) + g \mu_{\rm B} (B+B_{\rm A}) s_{\mathbf m}^+ . \nonumber\\ i \hbar \dfrac{d}{dt}t_{\mathbf m}^+ &= \left[ t_{\mathbf m} , \hat{H} \right] \label{dummy270224811}\\ &\stackrel{\rm (1st\, order)}{=} - 2 S \displaystyle\sum_{\mathbf r} J({\mathbf r}) (t_{\mathbf m}^+ + s_{\mathbf m+r}^-) \nonumber\\ &\quad- 2 S \displaystyle\sum_{\mathbf R} J_1({\mathbf R}) (-t_{\mathbf m}^+ + t_{\mathbf m+R}^+) + g \mu_{\rm B} (B+B_{\rm A}) t_{\mathbf m}^+ . \nonumber \end{align}
This calculation differs from that of the ferromagnet, in Inelastic magnetic scattering, by the complication that the equations of motion for the \(s^+\) operators involve the \(t^-\) operators and vice versa. Also the \(t^+\) operators and the \(s^-\) operators are mixed (not shown). Below, we show how to deal with this complication.
*Fourier and Boguliobov transformations
We now solve these two coupled equations of motion, describing the spins in the two sublattices. Firstly, Fourier transformation gives[3]
\begin{align} i \hbar \dfrac{d}{dt} S_{\mathbf q}^+ &= \left[ 2 S (J(0)-J_1(0)+J_1({\mathbf q})) + g \mu_{\rm B} (B+B_{\rm A})\right] S_{\mathbf q}^+ \label{dummy567299487}\\ &\quad + 2 S J({\mathbf q}) T_{\mathbf q}^- , \nonumber\\ i \hbar \dfrac{d}{dt} T_{\mathbf q}^- &= \left[ 2 S (-J(0)+J_1(0)-J_1({\mathbf q})) + g \mu_{\rm B} (B+B_{\rm A})\right] T_{\mathbf q}^- \label{dummy635732318}\\ &\quad - 2 S J({\mathbf q}) S_{\mathbf q}^+ .\nonumber \end{align}
Secondly, we perform a Boguliobov transformation, which is a linear transformation of two operators:
\begin{align} S_{\mathbf q}^+ &= u_{\mathbf q} \hat\alpha_{\mathbf q} + v_{\mathbf q} \hat\beta_{\mathbf q}^\dagger , \label{dummy36441344}\\ T_{\mathbf q}^- &= u_{\mathbf q} \hat\beta_{\mathbf q}^\dagger + v_{\mathbf q} \hat\alpha_{\mathbf q} .\label{dummy1987763760} \end{align}
The idea is that by a correct choice of the real coefficients \(u_{\bf q}\) and \(v_{\bf q}\), the new Boson operators, \(\hat\alpha_{\bf q}\) and \(\hat\beta_{\bf q}\) will perform independent oscillations. To determine the coefficients \(u_{\bf q}\) and \(v_{\bf q}\), we seek a number of boundary conditions. Firstly, Boson operators must fulfill the commutation relations:
\begin{equation} \label{dummy1071622307} [\hat\alpha_{\mathbf q}^\dagger,\hat\alpha_{\mathbf q'}] =\delta_{\mathbf q,q'} , \quad [\hat\beta_{\mathbf q}^\dagger,\hat\beta_{\mathbf q'}] =\delta_{\mathbf q,q'} . \end{equation}
Secondly, the "old" spin commutator relations must still be valid:
\begin{equation} \label{dummy2118875371} [ s_{\mathbf m}^+, s_{\mathbf m'}^- ] = 2s_{\mathbf m}^z \delta_{\mathbf m,m'} , \quad [ t_{\mathbf n}^+, t_{\mathbf n-}^- ] = 2t_{\mathbf n}^z \delta_{\mathbf n,n'} . \end{equation}
The resulting calculations are straightforward but tedious, and we will not list them here. We will proceed directly to the result for the energies.
*The spin wave energy
The antiferromagnetic spin waves have two branches, and their energies are[4]
\begin{align} \hbar \omega_0({\mathbf q}) &= + g \mu_{\rm B} B + \Omega({\mathbf q}) , \label{eq:omega_nonzerofield}\\ \hbar \omega_1({\mathbf q}) &= - g \mu_{\rm B} B + \Omega({\mathbf q}) ,\label{dummy196633099} \end{align}
where the zero field energy is
\begin{equation}\label{eq:omega_zerofield} \Omega({\mathbf q}) = \sqrt{\left[2SJ({\mathbf 0})-2S \left(J_1({\mathbf 0})-J_1({\mathbf q}) \right) + g \mu_{\rm B} B_{\rm A} \right]^2 - (2S)^2 J({\mathbf q})^2} . \end{equation}
The coefficients for the Boguliobov transformation are given by[5]
\begin{align} u_{\mathbf q}^2 - v_{\mathbf q}^2 &= 2 S , \label{dummy1668449917}\\ u_{\mathbf q}^2 &= 2S \; \dfrac{\Omega({\mathbf q})+2SJ({\mathbf 0})-2S[J_1({\mathbf 0})-J_1({\mathbf q})]+g \mu_{\rm B}B_{\rm A}} {2\Omega({\mathbf q})} ,\label{dummy210595604} \end{align}
leading to a few helpful expressions we will need later to determine the scattering cross section:
\begin{align} u_{\mathbf q}^2 + v_{\mathbf q}^2 &= 2S \; \dfrac{2SJ({\mathbf 0})-2S[J_1({\mathbf 0})-J_1({\mathbf q})]+g \mu_{\rm B}B_{\rm A}} {\Omega({\mathbf q})} , \label{dummy52712440}\\ u_{\mathbf q} v_{\mathbf q} &= \dfrac{-(2S)^2 J({\mathbf q})}{2 \Omega({\mathbf q})} .\label{dummy228124291} \end{align}
Example
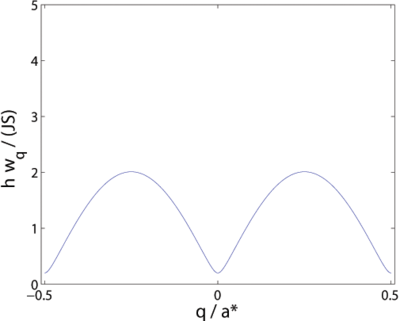
It is worth contemplating equation \eqref{eq:omega_nonzerofield}. Let us consider the simple case of nearest neighbour couplings only, \(J_1({\bf q})=0\), zero applied field, \(B=0\), and a zero anisotropy, \(B_{\rm A}=0\). Then, the spin wave energy becomes
\begin{equation} \label{dummy875378601} \hbar \omega_{\rm simple} = 2 S \sqrt{J(0)^2-J({\mathbf q})^2} . \end{equation}
For small values of \(|{\bf q}|\), this can be approximated by a linear dispersion of the form \(\hbar\omega = c |{\bf q}|\), where \(c\) is called the spin wave velocity. With a small anisotropy, the spin wave energy becomes
\begin{equation} \hbar \omega_{\rm simple, anis} = 2 S \sqrt{(J(0)+g\mu_{\rm B}B_{\rm A}/(2S) )^2-J({\bf q}')^2} , \end{equation}
as illustrated in Figure xx--CrossReference--fig:omegaq_AFM--xx.
*Quantum magnetism
Solving the Hamiltonian quantum mechanically is an easy task for a ferromagnet. Let us first rewrite the spin product term:
\begin{equation} {\bf S}_i \cdot {\bf S}_j = s_i^z s_j^ z + \frac{1}{2} (s_i^+ s_j^- + s_i^- s_j^+) , \end{equation}
where the spin raising and lowering operators are defined as
\begin{align} \label{eq:spinraising} s_{j}^+ &= s_{j}^x + i s_{j}^y , \\ s_{j}^- &= s_{j}^x - i s_{j}^y . \end{align}
The raising/lowering operators have the effect of changing \(m\), the eigenvalue of \(s^z\) for one spin, by one unit:
\begin{align} s_{j}^+ |S,m\rangle_j &= \sqrt{(S-m)(S+m+1)} |m+1\rangle_j , \\ s_{j}^- |S,m\rangle_j &= \sqrt{(S-m+1)(S+m)} |m-1\rangle_j . \end{align}
For completeness, we mention the commutator relations
\begin{align} \label{eq:commute_pm} [ s_j^+ , s_{j'}^- ] &= 2 s_j^z \delta_{j,j'} , \\{} [ s_j^z , s_{j'}^\pm ] &= \pm s_j^\pm \delta_{j,j'} , \\{} [ s_j^x , s_{j'}^y ] &= i s_j^z \delta_{j,j'} \; {\rm and\, cyclic\, permutations.} \end{align}
We can see that when using the Hamiltonian from the Elastic magnetic scattering page on the ferromagnetic state, \(|{\rm FM}\rangle = |\uparrow \uparrow \uparrow \uparrow \rangle\), all spin terms containing \(s^+\) vanish, leaving only the diagonal \(s^z_i s^z_j\) terms.
\begin{equation} H | {\rm FM} \rangle = - \sum_{\langle ij \rangle} J_{ij} S^2 | {\rm FM} \rangle . \end{equation}
The ferromagnetic state is thus an eigenstate of the Hamiltonian, and is also the ground state. However, for antiferromagnetic couplings, the Hamiltonian is a very difficult many-body problem. This can be seen from just considering the two-spin model for spins \(S=1/2\). Here, the classical ground states are \(| {\rm AFM}_1 \rangle = | \uparrow \downarrow \rangle\) and \(| {\rm AFM}_2 \rangle = | \downarrow \uparrow \rangle\). None of these states are eigenstates to the Hamiltonian, since terms in the Hamiltonian of the type \(s_1^+ s_2^-\) mix the states:
\begin{align} H | {\rm AFM}_1 \rangle &= - \frac{J}{4} |{\rm AFM}_1 \rangle + \frac{J}{2} |{\rm AFM}_2 \rangle , \\ H | {\rm AFM}_2 \rangle &= + \frac{J}{2} |{\rm AFM}_1 \rangle - \frac{J}{4} |{\rm AFM}_2 \rangle . \end{align}
The resulting ground state is a spin singlet:
\begin{equation} |{\rm GS}\rangle = \frac{1}{\sqrt{2}} \left({|\rm AFM}_1 \rangle - |{\rm AFM}_2 \rangle \right) , \end{equation}
with a ground state energy of \(-3J/4\), three times lower than (the expectation value of) the energy of the classical solution.
The antiferromagnetic quantum Heisenberg model for macroscopic systems has only been solved in a few simple one-dimensional cases, and only for spin values \(S=1/2\) and \(S=1\). The main conclusion is that there is no magnetic order in the classical sense, and that the ground state is a many-body quantum singlet state with short-range order. Three-dimensional systems are generally well described by a classical state with few modifications, whereas two-dimensional systems may show intermeditate (and highly interesting) behaviour, where the ground state could be described by a mixture of classical long-range order and quantum short-range order.
Magnetic quantum effects are revealed by the strength of the magnetic order, which is often smaller than expected classically (or completely absent). However, the fingerprint of quantum magnetism is found by inelastic magnetic neutron scattering.
Perspectives
Interest in magnetic quantum systems comes from the study of basic many-body quantum mechanics, entanglement of quantum systems with possible applications in quantum computing, in the study of zero-temperature "quantum phase transitions", as models for the string theory description of elementary particles, and as a basis for the study of correlated electron materials like the "heavy-fermion" systems and the high-temperature superconductors.
- ↑ P.W. Anderson. Phys. Rev., vol. 86, p. 694-701 (1952)
- ↑ W. Marshall and S.W. Lovesey. Theory of Thermal Neutron Scattering (Oxford, 1971), equation (9.222)
- ↑ W. Marshall and S.W. Lovesey. Theory of Thermal Neutron Scattering (Oxford, 1971), equation (9.227)
- ↑ W. Marshall and S.W. Lovesey. Theory of Thermal Neutron Scattering (Oxford, 1971), equation (9.234)
- ↑ W. Marshall and S.W. Lovesey. Theory of Thermal Neutron Scattering (Oxford, 1971), equation (9.230)
*Inelastic magnetic neutron scattering
In this section we will specialize the master equation for magnetic scattering to describe spin waves. We start with repeating the magnetic scattering master equation from the Magnetic neutron scattering page:
\begin{align}\label{dummy1597252741} \dfrac{d^2\sigma}{d\Omega dE_{\rm f}} &= \left(\gamma r_0 \right)^2 \dfrac{k_{\rm f}}{k_{\rm i}} \left[ \dfrac{g}{2} F(q)\right]^2 \displaystyle\sum_{\alpha \beta} \left( \delta_{\alpha\beta}-\hat{ {\mathbf q}}_\alpha\hat{ {\mathbf q}}_\beta\right) \\ &\quad \times \displaystyle\sum_{\lambda_{\rm i} \lambda_{\rm f}} p_{\lambda_{\rm i}} \displaystyle\sum_{j,j'} \left\langle \lambda_{\rm i}| \exp(-i {\mathbf q} \cdot {\mathbf r}_{j}) {\mathbf s}_{j}^\alpha | \lambda_{\rm f}\right\rangle \left\langle \lambda_{\rm f}\left| \exp(i {\mathbf q} \cdot {\mathbf r}_{j'}) {\mathbf s}_{j'}^\beta \right| \lambda_{\rm i}\right\rangle \nonumber\\ &\quad \times \delta\left( \hbar\omega + E_{\lambda_{\rm i}}-E_{\lambda_{\rm f}}\right) . \nonumber \end{align}
The next step closely follows the treatment of phonon scattering. First, we rewrite the energy-conserving \(\delta\)-function in terms of a time integral. To repeat the equation from the Scattering from lattice vibrations page:
\begin{equation}\label{eq:delta_transform} \delta(\hbar\omega+E_{\lambda_{\rm i}}-E_{\lambda_{\rm f}}) = \dfrac{1}{2\pi\hbar} \displaystyle\int_{-\infty}^{\infty} dt \exp\left(-\dfrac{i(\hbar\omega+E_{\lambda_{\rm i}}-E_{\lambda_{\rm f}})t}{\hbar}\right) . \end{equation}
Subsequently, we transform the stationary operators, here denoted \(\hat{A}\), to time-dependent Heisenberg operators, \(\hat{A}(t)\), through
\begin{align}\label{dummy1091740695} &\biggr\langle \lambda_{\rm i} \biggr| \exp\biggr( \dfrac{i E_{\lambda_{\rm i}} t}{\hbar} \biggr) \hat{A} \exp\biggr( -\dfrac{i E_{\lambda_{\rm f}} t}{\hbar} \biggr) \biggr| \lambda_{\rm f} \biggr\rangle \\ &\quad= \biggr\langle \lambda_{\rm i} \biggr| \exp\biggr( \dfrac{i \hat{H}_\lambda t}{\hbar} \biggr) \hat{A} \exp\biggr( -\dfrac{i \hat{H}_\lambda t}{\hbar} \biggr) \biggr| \lambda_{\rm f} \biggr\rangle \nonumber\\ &\quad= \langle \lambda_{\rm i} | \hat{A}(t) | \lambda_{\rm f} \rangle .\nonumber \end{align}
Making this transformation and performing the closure sum over the final states, (\(\sum_{\lambda_{\rm f}}|\lambda_{\rm f}\rangle\langle\lambda_{\rm f}| = 1\)), we reach the equation for the magnetic scattering cross section in the Heisenberg picture[1]:
\begin{align}\label{dummy72416411} \dfrac{d^2\sigma}{d\Omega dE_{\rm f}} &= \left(\gamma r_0 \right)^2 \dfrac{k_{\rm f}}{k_{\rm i}} \left[ \dfrac{g}{2} F(q)\right]^2 \displaystyle\sum_{\alpha \beta} \left( \delta_{\alpha\beta}-\hat{q}_\alpha\hat{q}_\beta\right) \\ &\quad\times \dfrac{1}{2\pi\hbar} \displaystyle\sum_{j,j'} \displaystyle\int_{-\infty}^{\infty} dt \exp(-i \omega t) \nonumber\\ &\quad\times \left\langle \exp(-i {\mathbf q} \cdot {\mathbf r}_{j}(0)) {\mathbf s}_{j}^\alpha(0) \exp(i {\mathbf q} \cdot {\mathbf r}_{j'}(t)) {\mathbf s}_{j'}^\beta(t) \right\rangle , \nonumber \end{align}
where \(\langle \hat{A} \rangle\) means the thermal average of the expectation value of an operator \(\hat{A}\), in the same way as the calculations leading to the phonon expression on the Scattering from lattice vibrations page.
The nuclear positions \({\bf r}(t)\) and the atomic spin \({\bf s}(t)\) are both operators. Each of these give rise to both an elastic and an inelastic contribution; in total the cross section becomes a sum of four terms. The one that is elastic in both channels is the magnetic diffraction signal, while the contribution that is elastic in the nuclear positions (the phonon channel) and inelastic in the spins (the magnetic channel) is the true magnetic inelastic signal. The two other contributions involve creation/annihilation of phonons via the magnetic interactions, and are not discussed further here; we refer to Ref. [2].
The two contributions that are elastic in the phonon channel are reached in analogy with the page Scattering from lattice vibrations. We replace the general nuclear position operators \({\bf r}_j\) with the nuclear equilibrium positions in a lattice and utilize the periodicity of the lattice. Furthermore, we multiply with the Debye-Waller factor, \(\exp(-2W)\)[3]:
\begin{align} \label{eq:magn_cross_master} \left(\dfrac{d^2\sigma}{d\Omega dE_{\rm f}}\right)_{\rm magn.} &= \left(\gamma r_0 \right)^2 \dfrac{k_{\rm f}}{k_{\rm i}} \left[ \dfrac{g}{2} F(q)\right]^2 \exp(-2W) \displaystyle\sum_{\alpha \beta} \left( \delta_{\alpha\beta}-\hat{ {\mathbf q}}_\alpha\hat{ {\mathbf q}}_\beta\right) \\ &\quad\times N \dfrac{1}{2\pi\hbar} \displaystyle\int_{-\infty}^{\infty} dt \exp(-i \omega t) \displaystyle\sum_{j'} \exp(i {\mathbf q} \cdot ({\mathbf r}_j)) \left\langle {\mathbf s}_{0}^\alpha(0) {\mathbf s}_{j'}^\beta(t) \right\rangle . \nonumber \end{align}
This equation should be understood as the space and time Fourier transform of the spin-spin correlation function, and it is the starting point for most calculations of magnetic scattering cross sections.
- ↑ W. Marshall and S.W. Lovesey. Theory of Thermal Neutron Scattering (Oxford, 1971), equation (8.2)
- ↑ W. Marshall and S.W. Lovesey. Theory of Thermal Neutron Scattering (Oxford, 1971), equation (8.10)
- ↑ W. Marshall and S.W. Lovesey. Theory of Thermal Neutron Scattering (Oxford, 1971), equation (8.13) and (8.25)
*Neutron cross section from ferromagnetic spin waves
For calculating the neutron scattering cross section for ferromagnetic spin waves, we begin with the master equation \eqref{eq:magn_cross_master} and use the description of spin waves above.
We describe the spin-spin correlation functions in terms of the "coordinates" \(+\), \(-\) and \(z\). The sum of the \(z\)-component of the spins, \(S^z=\sum_{j}s_{j}^z\), commute with \(\hat{H}\), making this sum a constant of motion. Hence, the number of \(+\) and \(-\) operators must be the same for the operator to have an expectation value. It is then easy to see that spin correlation functions of the types \(\langle s^+(0) s^+(t) \rangle\) and \(\langle s^+(0) s^z(t)\rangle\) vanish, leaving only three non-zero terms: \(\langle s^z(0) s^z(t)\rangle\), \(\langle s^+(0) s^-(t) \rangle\), and \(\langle s^-(0) s^+(t) \rangle\). Since \(S^z\) is a constant of motion, we have \(s_{j}^z(t)=s_{j}^z(0)\), and hence the \(zz\) term gives rise to elastic scattering.
The spin wave cross section comes from the term
\begin{align}\label{dummy132877240} \langle s_{j}^-(0) s_{j'}^+(t) \rangle &= \dfrac{1}{N} \displaystyle\sum_{\mathbf q'} \exp(i{\mathbf q'}\cdot ({\mathbf r}_{j'}-{\mathbf r}_j)) \langle S_{\mathbf q'}^-(0) S_{\mathbf q'}^+(t) \rangle \\ &= \dfrac{2\langle S^z \rangle}{N} \displaystyle\sum_{\mathbf q'} \exp(i{\mathbf q'}\cdot ({\mathbf r}_{j'}-{\mathbf r}_{j})) \exp(-i \omega_{\mathbf q'}t) n_{\rm B}\biggr( \frac{\hbar \omega_{\mathbf q'}}{k_{\rm B}T} \biggr) .\nonumber \end{align}
We note the value of the expectation value \(\langle S_{\bf q'}^- S_{\bf q'}^+ \rangle = 2 \langle S^z \rangle n_{\rm B}(\hbar\omega_{\bf q'}/k_{\rm B}T)\), where the thermal population factor for Bosons is given by this equation on the Scattering from lattice vibrations page.
Since \([S_{\bf q'}^+,S_{\bf q'}^-]=2 S^z\), we immediately have
\begin{align}\label{dummy1302615768} \langle s_{j}^+(0) s_{j'}^-(t) \rangle &= \dfrac{1}{N} \displaystyle\sum_{\mathbf q'} \exp(-i{\mathbf q'}\cdot ({\mathbf r}_{j'}-{\mathbf r}_j)) \langle S_{\mathbf q'}^-(0) S_{\mathbf q'}^+(t) + 2 S^z \rangle \\ &= \dfrac{2 \langle S^z \rangle}{N} \displaystyle\sum_{\mathbf q'} \exp(-i{\mathbf q'}\cdot ({\mathbf r}_{j'}-{\mathbf r}_j)) \exp(i \omega_{\mathbf q'}t) \biggr( n_{\rm B}\biggr( \frac{\hbar\omega_{\mathbf q'}}{k_{\rm B}T}\biggr)+1\biggr) . \nonumber \end{align}
To transform back to the \(x\) and \(y\) operators, we note that the \(x\) and \(y\) axes are equivalent, whence \(\langle s_{j}^x(0)s_{j'}^x(t)\rangle = \langle s_{j}^y(0)s_{j'}^y(t)\rangle\). Moreover, the sum of the two mixed terms \(\langle s_{j}^x(0)s_{j'}^y(t) + s_{j}^y(0)s_{j'}^x(t)\rangle\) is seen to be zero. We calculate directly
\begin{equation} \label{dummy1512931648} \langle s_{j}^x(0)s_{j'}^x(t)\rangle = \dfrac{1}{4}\langle s_{j}^+(0)s_{j'}^-(t) + s_{j}^-(0) s_{j'}^+(t) \rangle . \end{equation}
This allows us to calculate the coordinate sum entering the cross section expression:
\begin{align}\label{dummy1389285453} \displaystyle\sum_{\alpha\beta} \left( \delta_{\alpha\beta}-\hat{q}_\alpha\hat{q}_\beta\right) \langle s_{j}^\alpha(0)s_{j'}^\beta(t)\rangle &= (2-\hat{q}_x^2-\hat{q}_y^2) \langle s_{j}^x(0)s_{j'}^x(t)\rangle \\ &= \dfrac{1}{4} (1+\hat{q}_z^2) \langle s_{j}^+(0)s_{j'}^-(t) + s_{j}^-(0)s_{j'}^+(t) \rangle .\nonumber \end{align}
Inserting into equation \eqref{eq:magn_cross_master}, the expression for the inelastic neutron scattering cross section for a ferromagnet reads:
\begin{align}\label{dummy2135302037} \left( \dfrac{d^2\sigma}{d\Omega dE_{\rm f}}\right)_{\text{magn.}} &= \left(\gamma r_0 \right)^2 \dfrac{k_{\rm f}}{k_{\rm i}} \left[ \dfrac{g}{2} F(q)\right]^2 \exp(-2W)\left( 1+\hat{q}_z^2 \right) \dfrac{\langle S^z \rangle}{2} \\ &\quad\times \dfrac{(2\pi)^3}{v_0} \displaystyle\sum_{ {\mathbf q},{\boldsymbol\tau}} \biggr[ \biggr(n_{\text{B}}\biggr( \frac{\hbar\omega_{\mathbf q}}{k_{\text{B}}T} \biggr) +1\biggr) \delta\left(\hbar\omega_{\mathbf q'}-\hbar\omega \right) \delta\left( {\mathbf q'} - {\mathbf q} - {\boldsymbol\tau} \right) \nonumber\\ &\qquad\qquad\qquad\quad + n_{\text{B}}\biggr( \frac{\hbar\omega_{\mathbf q'}}{k_{\text{B}}T} \biggr) \delta\left(\hbar\omega_{\mathbf q'}+\hbar\omega \right) \delta\left( {\mathbf q'} + {\mathbf q} - {\boldsymbol\tau} \right) \biggr], \nonumber \end{align}
where \({\boldsymbol\tau}\) is a reciprocal lattice vector and \(v_0\) is the volume of the unit cell. We can see that the cross section depends upon energy and temperature only through the thermal population factor, \(n_{\rm B}\), and the ordered moment, \(\langle S^z \rangle\). At low temperatures, compared to the spin wave energy, the spin wave spectral weight is constant, apart from the slow variation with \(q\) of the magnetic structure factor, \(F(q)\), and the Debye-Waller factor, \(\exp(-2W)\). Notice that the spin wave intensity is highest when \({\bf q}\) is in the direction of the ordered moment. This can be understood by the spin wave being a purely transverse excitation.
*Neutron cross section of antiferromagnetic spin waves
For the calculation of the neutron scattering cross section for antiferromagnetic spin waves, we again invoke the master equation \eqref{eq:magn_cross_master} and use the solutions for the antiferromagnetic spin waves above.
Firstly, we calculate the correlation functions. As for the ferromagnetic spin waves, the \(zz\) term is elastic and other terms involving \(z\) vanish. also the \(++\) and \(--\) terms vanish. Hence, we need only to calculate
\begin{align} \left\langle S_{\mathbf q}^- S_{\mathbf q}^+ \right\rangle &= \left\langle (u_{\mathbf q} \hat\alpha_{\mathbf q}^+ + v_{\mathbf q}\hat\beta_{\mathbf q}) (u_{\mathbf q}\hat\alpha_{\mathbf q} + v_{\mathbf q}\beta_{\mathbf q}^+) \right\rangle \label{dummy141100595}\\ &= u_{\mathbf q}^2 n_{\mathbf q} + v_{\mathbf q}^2 (n_{\rm B}(\hbar\omega_{\mathbf q}/k_{\rm B}T)+1) .\label{dummy1267455531} \end{align}
Transforming to real space and \(x-y\) coordinates, the final expression reads:
\begin{align}\label{dummy785616823} \left( \dfrac{d^2\sigma}{d\Omega dE_{\rm f}}\right)_{\rm magn.} &= \left(\gamma r_0 \right)^2 \dfrac{k_{\rm f}}{k_{\rm i}} \left[ \dfrac{g}{2} F(q)\right]^2 \exp(-2W)\left( 1+\hat{q}_z^2 \right) \dfrac{1}{4} \dfrac{(2\pi)^3}{v_0} \\ &\quad \times \displaystyle\sum_{ {\mathbf q},{\boldsymbol\tau} ,a} \biggr[ \biggr(n_{\rm B}\biggr( \dfrac{\hbar\omega_{a, \mathbf q}}{k_{\rm B}T} \biggr) +1\biggr) \delta\left(\hbar\omega_{\mathbf q'}-\hbar\omega \right) \delta\left( {\mathbf q'} - {\mathbf q} - {\boldsymbol\tau} \right) \nonumber\\ &\qquad\qquad\qquad + n_{\rm B}\biggr( \dfrac{\hbar\omega_{a, \mathbf q}}{k_{\rm B}T} \biggr) \delta\left(\hbar\omega_{\mathbf q'}+\hbar\omega \right) \delta\left( {\mathbf q'} + {\mathbf q} - {\boldsymbol\tau} \right) \biggr] \nonumber\\ &\quad \times \left[ u_{\mathbf q}^2 + v_{\mathbf q}^2 + 2 u_{\mathbf q} v_{\mathbf q} \cos({\boldsymbol\rho} \cdot {\boldsymbol\tau}) \right] , \nonumber \end{align}
where \({\boldsymbol\tau}\) is a reciprocal lattice vector of a sublattice, \({\boldsymbol\rho}\) is a vector joining the sublattices, and \(a\) is the sublattice index. The difference between this cross section and the one for ferromagnetic spin waves is the presence of the coherence factor, \(u_{\bf q}^2 + v_{\bf q}^2 + 2 u_{\bf q} v_{\bf q} \cos({\boldsymbol\rho} \cdot {\boldsymbol\tau})\). It should be noted that the term \(\cos({\boldsymbol\rho} \cdot {\boldsymbol\tau})\) assumes the value 1 for \({\boldsymbol\tau}\) being a nuclear peak and \(-1\) for \({\boldsymbol\tau}\) being an AFM peak.
We now proceed to calculate the cross section in a few simple cases.
*The simple nearest neighbour antiferromagnet
In some lattice types, like the bcc lattice, all nearest neighbours are ions from the opposite sublattice. Hence, for a nearest neighbour system, \(J_1({\bf q})=0\). We assume absence of anisotropy, \({\bf B}_{\rm A}=0\). Hence, the expressions simplify to
\begin{align} \Omega_{\mathbf q} &= 2S \sqrt{J({\mathbf 0})^2 - J({\mathbf q})^2} , \label{dummy235263662}\\ u_{\mathbf q}^2 + v_{\mathbf q}^2 &= \dfrac{(2S)^2 J({\mathbf 0})}{\Omega_{\mathbf q}} , \label{dummy983085806}\\ 2 u_{\mathbf q} v_{\mathbf q} &= \dfrac{-(2S)^2 J({\mathbf q})}{\Omega_{\mathbf q}} .\label{dummy80770084} \end{align}
For scattering close to an AFM peak (\(\cos({\boldsymbol\rho} \cdot {\boldsymbol\tau})=-1\)), the coherence factor can be written as
\begin{equation}\label{eq:coh_simple} \left[ u_{\mathbf q}^2 + v_{\mathbf q}^2 + 2 u_{\mathbf q} v_{\mathbf q} \cos({\boldsymbol\rho} \cdot {\boldsymbol\tau}) \right] = 2 S \sqrt{\dfrac{J({\mathbf 0})+J({\mathbf q})}{J({\mathbf 0})-J({\mathbf q})}} , \end{equation}
which diverges as \(1/\Omega_{\bf q}\) for low values of \(|{\bf q}|\). Close to a nuclear Bragg peak (\(\cos({\boldsymbol\rho} \cdot {\boldsymbol\tau})=1\)), the solution is the reciprocal of equation \eqref{eq:coh_simple}, having a value of zero for \(|{\bf q}|=0\). At the zone boundary, \(J({\bf q})=0\), and the coherence factor equals \(2S\).
*Antiferromagnetic nanoparticles in zero field
In nanoparticles, the spin wave spectrum is quantized. However, only the \({\bf q}=0\) mode has ever been observed[1]. The energy of the lowest mode, the collective magnetic precessions, is thus completely determined by the anisotropy:
\begin{align} \Omega_{\mathbf 0} &= g \mu_{\rm B} B_{\rm A} ,\label{dummy728084531}\\ u_{\mathbf 0}^2 + v_{\mathbf 0}^2 &= \dfrac{(2S)^2 J({\mathbf 0})+g \mu_{\rm B} B_{\rm A}} {\Omega_{\mathbf 0}} ,\label{dummy2008801156}\\ 2 u_{\mathbf 0} v_{\mathbf 0} &= \dfrac{-(2S)^2 J({\mathbf 0})}{\Omega_{\mathbf 0}} .\label{dummy597972028} \end{align}
For \({\boldsymbol\tau}\) being an AFM Bragg peak, \(\cos({\boldsymbol\rho} \cdot {\boldsymbol\tau})=-1\), the coherence factor is approximately
\begin{equation}\label{eq:coh_nano} \left[ u_{\mathbf 0}^2 + v_{\mathbf 0}^2 + 2 u_{\mathbf 0} v_{\mathbf 0} \cos({\boldsymbol\rho} \cdot {\boldsymbol\tau}) \right] \approx 2 S \dfrac{2 J({\mathbf 0})}{g \mu_{\rm B} B_{\rm A}} , \end{equation}
which usually is a very large number. On the other hand, for \({\boldsymbol\tau}\) being a nuclear Bragg peak, \(\cos({\boldsymbol\rho} \cdot {\boldsymbol\tau})=1\), the coherence factor equals unity, much lower than for the AFM Bragg peak. This explains why collective magnetic excitations are not observed at the position of nuclear Bragg peaks.
- ↑ M.F. Hansen et al.. Phys. Rev. Lett., vol. 79, p. 4910-4913 (1997)
← Previous page: Elastic magnetic scattering
→ Next page: Monte Carlo simulation of neutron instrumentation